Asteroid families
are groups of asteroids that are supposed to have a common origin in
the collisional event that shattered the parent body. They are usually
determined by identifying clusters of objects close in proper elements
domain (a,e,sin(i)). The Hierarchical Clustering Method (HCM
hereafter) as described by Bendjoya and Zappalŕ (2002) operates by
identifying all objects that are closer than a given distance (cutoff)
with respect to at least one other member of a family.
Recently the Sloan Digital Sky Survey-Moving Object Catalog data,
fourth release (Ivezic et al. 2002, SDSS-MOC4), provided multi-band
photometry on a sample two order of magnitude larger than any available
in any current spectroscopic catalogs (about 60000 numbered objects).
Parker et al. (2008) constructed a color-code diagram in a (a', i-z) plane where:
and C1, C2, and C3 are numerical coefficients that depend on the color values, on the number of observations in the given database (Roig and Gil-Hutton 2006), and SDSS colors, known with an accuracy of about 0.03 magnitudes. Bus and Binzel (2002a,b) and Nesvorný et al. (2005) found asteroid families in extended domains of proper elements and SDSS-MOC4 principal components data in order to minimize the number of possible interlopers, but such an analysis was not extended to asteroids' geometrical albedo. Asteroid taxonomies are however also determined by the body geometric albedo: C-type asteroids tend to have lower values of geometric albedo than S-type ones.
Tholen asteroid taxonomy used values of pV to distinguish classes of asteroids inside the X-complex, such as the M-, E-, and P-types. Until recently, however, only about two thousand asteroids had reliable values of geometric albedos (see Tedesco et al. 2002). Wide-field Infrared Survey Explorer (WISE) (Wright et al. 2010), and the NEOWISE (Mainzer et al. 2011) enhancement to the WISE mission allowed to obtain diameters and geometric albedo values for more than 100,000 Main Belt asteroids (Masiero et al. 2011), increasing the sample of objects for which albedo values were known by a factor 50. In this work we propose a new method quite effective in determining objects belonging to a family halo, with low percentages of likely interlopers, and results that are quite consistent in term of taxonomy and geometric albedo of the halo members. We take full advantage of the newly available WISE data and we introduce a new hierarchical clustering method in a multi-domain space composed by asteroids proper elements (a,e,sin(i)), SDSS-MOC4 colors (a*,i-z), and WISE geometric albedo pV, to identify halos associated with main belt asteroid families.
To avoid including too many interlopers in the family halo we proposed to work with a metric in a multi-domain space composed by proper elements (a,e,sin(i)), SDSS-MOC4 colors (a*,i-z), and WISE geometric albedo pV, to identify halos associated with main belt asteroid families. We eliminated from our sample asteroids with errors in : a* or (i-z) larger than 0.1 magnitudes, and with pV larger than 0.05 if pV < 0.2, and pV larger than 0.1 if pV > 0.2. We also eliminated objects in the inner main belt with pV > 0.5, since these objects may have large errors in albedos associated with the way absolute magnitude are calculated (Masiero et al. 2011). We then introduced a distance metric in this multi-domain space defined as:
where d is the standard distance metric in proper element domain (Zappalá et al. 1995), δa* = a*2 – a*1, and similar relationships hold for δ(i-z) and δpV. CSPV is a weighting factor equal to 106.
Our result are summarized in the next web-pages. Click on the links in the table below to access the data on the five orbital regions that we studied. The tables in each of the five regions have links to the files with the information on the asteroid families that we studied, in the format # of family member, asteroid identification, a,e,sin(i),n,g,s, H, Ly, pV, δpV, a*, i-z, wher n is the proper mean-motion, g and s are the precession frequencies of the argument of pericenter and longitude of the node, H is the absolute magnitude, and Ly is the Lyapunov exponent.
Questions? Comments? We would like to hear from you. Please send us an email.
N.B. The links above will be made available after publication of the MNRAS article that describes our method.
| If an object is closer than this distance, it is associated to the
dynamical family, and the procedure is repeated until no new family
members are found. For small values of the cutoff only the objects
closest in proper element domain are identified as family members: the
family ``core''. At larger cutoff one is able to identify objects that,
while still belonging to the collisional group, may have dynamically
evolved since the family formation and driftedapart from the core: the
family ``halo''. One problem in obtaining a good determination of a family halo is however the presence of objects in the orbital region of the halo that might not be, for taxonomical reasons, associated with the local family: the interlopers. |
 |
| An analysis of spectral
properties of local asteroids may provide insights on the possible
presence of such interlopers in groups found in proper elements
domains, but such information is usually available only for 2% or less
of the main belt asteroids. |
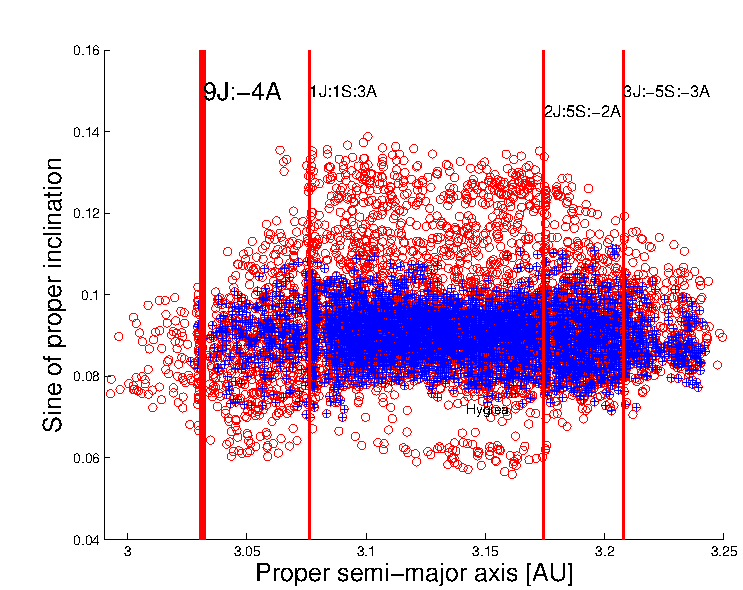
The figure shows an (a,sin(i)) projection of the Hygiea family core (blue crosses) and halo (red circles), as obtained in Carruba (2013). Vertical red lines displays the location of the main mean-motion resonances in the region. |
Parker et al. (2008) constructed a color-code diagram in a (a', i-z) plane where:
a*= C1 (g-r)+C2(r-i)+C3 (1)
and C1, C2, and C3 are numerical coefficients that depend on the color values, on the number of observations in the given database (Roig and Gil-Hutton 2006), and SDSS colors, known with an accuracy of about 0.03 magnitudes. Bus and Binzel (2002a,b) and Nesvorný et al. (2005) found asteroid families in extended domains of proper elements and SDSS-MOC4 principal components data in order to minimize the number of possible interlopers, but such an analysis was not extended to asteroids' geometrical albedo. Asteroid taxonomies are however also determined by the body geometric albedo: C-type asteroids tend to have lower values of geometric albedo than S-type ones.
Tholen asteroid taxonomy used values of pV to distinguish classes of asteroids inside the X-complex, such as the M-, E-, and P-types. Until recently, however, only about two thousand asteroids had reliable values of geometric albedos (see Tedesco et al. 2002). Wide-field Infrared Survey Explorer (WISE) (Wright et al. 2010), and the NEOWISE (Mainzer et al. 2011) enhancement to the WISE mission allowed to obtain diameters and geometric albedo values for more than 100,000 Main Belt asteroids (Masiero et al. 2011), increasing the sample of objects for which albedo values were known by a factor 50. In this work we propose a new method quite effective in determining objects belonging to a family halo, with low percentages of likely interlopers, and results that are quite consistent in term of taxonomy and geometric albedo of the halo members. We take full advantage of the newly available WISE data and we introduce a new hierarchical clustering method in a multi-domain space composed by asteroids proper elements (a,e,sin(i)), SDSS-MOC4 colors (a*,i-z), and WISE geometric albedo pV, to identify halos associated with main belt asteroid families.
To avoid including too many interlopers in the family halo we proposed to work with a metric in a multi-domain space composed by proper elements (a,e,sin(i)), SDSS-MOC4 colors (a*,i-z), and WISE geometric albedo pV, to identify halos associated with main belt asteroid families. We eliminated from our sample asteroids with errors in : a* or (i-z) larger than 0.1 magnitudes, and with pV larger than 0.05 if pV < 0.2, and pV larger than 0.1 if pV > 0.2. We also eliminated objects in the inner main belt with pV > 0.5, since these objects may have large errors in albedos associated with the way absolute magnitude are calculated (Masiero et al. 2011). We then introduced a distance metric in this multi-domain space defined as:
dmd={d2+CSPV [(δa*)2+( δ (i−z ))2+(δpV )2 ]}1/2 (2)
where d is the standard distance metric in proper element domain (Zappalá et al. 1995), δa* = a*2 – a*1, and similar relationships hold for δ(i-z) and δpV. CSPV is a weighting factor equal to 106.
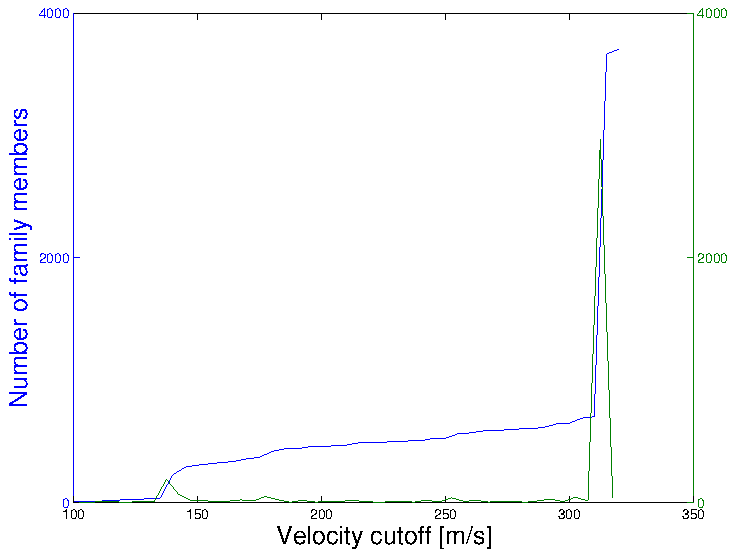
As first halo members, we selected asteroids that belong to the asteroids family, whose spectral type is compatible with that of the other members. We then obtained dynamical groups using Eq. (2), for a value of cutoff dmd a bit less than the value for which the family halo merges with the local background. The figure displays the number of members (blue line) and new members (green line) of the Themis group as a function of the velocity cutoff dmd. |
 |
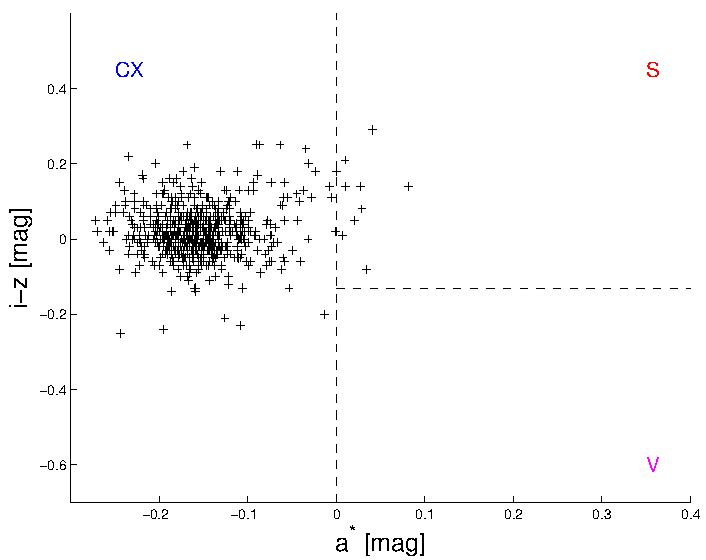
We then eliminated possible interlopers, defined as objects in regions of the (a',i-z) not appropriate for the family, and with albedo values outside the range usually expected (< 0.1 for CX-complex objects, > 0.1 for S-complex objects). The figure shows an (a*,i-z) projection of Themis family asteroids, with the division of the plane in the CX-complex, S-complex, and V-type asteroids areas. |
 |
Our result are summarized in the next web-pages. Click on the links in the table below to access the data on the five orbital regions that we studied. The tables in each of the five regions have links to the files with the information on the asteroid families that we studied, in the format # of family member, asteroid identification, a,e,sin(i),n,g,s, H, Ly, pV, δpV, a*, i-z, wher n is the proper mean-motion, g and s are the precession frequencies of the argument of pericenter and longitude of the node, H is the absolute magnitude, and Ly is the Lyapunov exponent.
| Region |
Number of detected families |
| Hungaria region |
2 |
| Inner main belt |
7 |
| Central main belt |
31 |
| Outer main belt |
19 |
| Cybele region |
3 |
Questions? Comments? We would like to hear from you. Please send us an email.
N.B. The links above will be made available after publication of the MNRAS article that describes our method.